A 2D Model of Georges Bank in the Summer, Forced Only By M2 Tides
This 2D model of Georges Bank was developed by Dr. Changsheng Chen of the University of Georgia, and has been described in Chen et al. (1995), Chen and Beardsley (1995), and Franks and Chen (1996). It is based on the Ecom-si code described in Blumberg and Mellor (1987). It is run with a uniform summertime stratification of 0.03 degrees Celsius per meter, and, in the movies below, has been forced with the M2 tides and run until the density field has reached a steady state (averaged over a tidal cycle). The model has 61 levels in the vertical, and has 1 km horizontal resolution. The vertical turbulence closure is a full level 2.5 Mellor Yamada scheme.
The particle tracking code used to produce the movies below were written by J. Pringle based on routines kindly provided by Changsheng Chen.
Playing the movies below:
The movies below are in the .flc format- Instruction for playing these movies can be found on the USGS web page.
If your link to the Internet is slow:
All of the movies and this page can be downloaded as a compressed tar file for those with slow links- this is much faster since the combined tar file is one-third the size of the files individually.
Movies of the Flow, Temperature, and Vertical Mixing Fields
The flow, temperature, and vertical mixing coefficients for temperature are shown below for four portions of the model domain:
Georges Bank Crest- Note the tidally modulated vertical mixing, and the vertical phase lags in the velocity fields caused by friction. These interact with the horizontal density gradients to create unstable stratification on the bank for part of each tidal cycle. [6.4Mbytes]
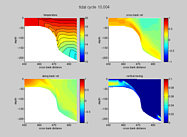Northern Flank- Note the strong vertical phase shifts in the cross bank velocity over the steepest part of the bank- These are driven by intense horizontal pressure gradients formed when less dense water is pulled off the bank by tides. These phase shifted currents lead to unstable stratification and strong vertical mixing over the steepest portions of the north flank. [The line contours in the plots of vertical diffusivity represent diffusivities of 0.25, 0.5 and 0.75 meters squared per second]. [3.8MBytes]
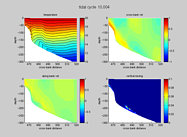Northern Flank, extended- Same as the above movie, but the region displayed has been shifted 35 km northward to better illustrate the generation of internal tides. [3.8Mbytes]
Southern Flank- Again, notice the internal tide generation and frictional and buoyancy driven vertical phase lags in the velocity fields and the tidally modulated mixing. [2.7Mbytes]
Movies of Float paths
The movies below show the paths of particles released in several columns over the bank. The floats are moved both by the mean currents and the turbulent velocities which are parameterized in the model by vertical diffusivities. The movies run for two tidal cycles.
Georges Bank Crest- Notice the horizontal dispersion of the particles caused by vertical mixing and horizontal shear flows. This leads to an effective horizontal diffusivity of 40-80 meters squared per second. [29Mbytes]
Northern Flank- Notice the cross-bank transport of particles in the bottom boundary layer over the steepest portion of the bank (Chen and Beardsley, In Press), and the shear/strain dispersion elsewhere in the bottom boundary layer. Also notice the lack of a net motion over a tidal cycle of particles in the well stratified regions. [29.3Mbytes]
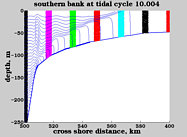Southern Flank- Note the difference in the horizontal dispersion of the particles in and out of the bottom boundary layers- The presence of vertical stratification inhibits vertical mixing and thus inhibits the shear/strain horizontal diffusion mechanism. Also notice the lack of any mean cross bank motion of particles in the bottom boundary layer. [8.7Mbytes]
Bibliography:
Blumberg, A.F. and G.L. Mellor. 1987. A Description of a Three-Dimensional Coastal Ocean Circulation Model. Three -Dimensional Coastal Ocean Models, N.S. Heaps, ed. Coast. and Estuarine Sci., 4, pp. 1-16
Chen, C. and R.C. Beardsley. 1995. A Numerical Study of Stratified Tidal Rectification Over a Finite-Amplitude Symmetric Bank. J. Phys. Oceanogr., 25, pp. 2090-2110
Chen, C., R.C. Beardsley., and R. Limeburner. 1995. A Numerical Study of Stratified Tidal Rectification Over Georges Bank. J. Phys. Oceanogr., 25, pp. 2111-2128
Franks, P. J. S. and C. Chen. 1996. Plankton Production in Tidal Fronts: A Model of Georges Bank in Summer. J. Mar. Res., 54, pp. 631-651
BCO-DMO Blog
©2024 Biological and Chemical Oceanography Data Management Office.
